Depth of field

Depth of field (usually abbreviated as DOF) is usually defined as the range of distances from the camera within which objects are rendered in focus in a photograph. Most photographic lenses with a manual focus ring and a distance scale also have markings that indicate its depth of field at one or more aperture settings (in the example below, both for f/16 and f/32).

The depth of field extends both forward and backward from the plane of maximum focus, and increases when stopping down a lens. It is also commonly stated that wide-angle lenses have a higher depth of field than telephoto lenses. A shallow depth of field makes it easier to focus manually on a subject, because out-of-focus details are blurrier. Therefore, focusing is generally performed with the diaphragm wide open and the depth of focus at its minimum (another reason for this is that it is easier to judge a bright picture in the viewfinder than a dark one).

If one looks at the laws of optics that govern the performance of lenses, however, the situation is different. Regardless of the numerical aperture of a lens, there is only a distance at which the subject is at maximum focus. Subjects that are located out of this plane are not in focus, and therefore blurry. The amount of blurriness increases with the distance of the subject from the plane of maximum focus. Thus, DOF is always zero in reality, and the concept of depth of field as applied in photography is based on the perception of what is sharp (i.e., blurry but to an acceptable degree) or unsharp (blurry to an unacceptable degree). There is also a continuous gradation between sharp and unsharp, and where to draw a borderline between the two states is therefore a matter of perception and accepted standards, not of physical laws.

Circle of confusion

The circle of confusion in a photograph is defined as the maximum size of an unsharp blob that is still perceived as a point, instead of a disc (given that this definition relies largely on subjective perception, someone defined "circle of confusion" as a group of photographers meeting to discuss the definition of DOF). The diameter of the circle of confusion depends of course on the size of the photograph and the distance of the observer: taking a well-focused picture on 35 mm negative film, printing it the size of a poster and looking at it from a distance of 20 cm will show everything grainy and blurry. Printing the same negative to the size of a typing-paper sheet and looking at it from arm's length will show the details in their sharpness. Therefore, an official standard is needed. Like all standards, it does not cover all possible circumstances, and instead is based on a range of circumstances judged to be frequent and usable in practice.

The standard for the circle of confusion assumes a print approximately 20 by 25 cm in size, viewed form a distance of 60 to 90 cm. On this print, a circle of confusion is 0.2 mm wide. A 35 mm negative is enlarged 8.3 times to make such a print, and the circle of confusion on the negative is roughly 0.025mm. This value is often approximated to 0.03 mm. If a digital image created by a current Nikon DSLR is used, the area of the sensor is half the area of a 35 mm film frame, i.e., approximately 16 by 24 mm. The circle of confusion is correspondingly smaller, at 0.017 mm (often approximated to 0.02 mm).

The above circle of confusion is generally used for low-end, everyday needs. A much smaller circle of confusion is used for high-end needs, like professional slides (typically, 0.02 mm for 35 mm film) or situations in which the resolution of the film must be fully utilized (0.01 mm for 35 mm film).

The depth of field D is computed as: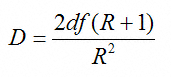

where d is the diameter of the circle of confusion, f is the aperture expressed as f/stop (i.e., focal length/diaphragm aperture: for example, 1/2.8 for a stop setting of 2.8), and R is the reproduction ratio. The first thing to notice is that the focal length of the lens does not appear anywhere in the formula. This means that focal length does not affect the depth of field. The above formula is a good approximation at long distances to the subject. A more precise formula useful at close range is provided here.

How do we reconcile the above statement with our unmistakable perception that wide-angle lenses do have a much higher depth of field than telephoto lenses? The explanation is actually very simple. In the above formula, it is obvious that R is very important in determining D. Thus, in order to compare the depth of field of lenses of varying focal lengths, we must use a subject of constant size, and shoot it at a constant reproduction ratio. Once we do this, it becomes apparent that, in these conditions, the depth of field is indeed independent of focal length. If you take a picture of a person with a wide-angle, you must compare it with a picture of the same person, reproduced on the film or sensor at the same size as the preceding picture, taken with a telephoto lens. In order to do this, you must walk quite a bit away from the subject in order to take an appropriate picture. Perspective effects, which change with focal length, are another factor involved. A wide-angle includes a proportionately larger background than a telephoto. This changes our perception of the depth of field (in particular, a larger amount of background tends to mask the unsharpness in its details, and therefore unsharpness is more evident when a telephoto is used).

The second thing to notice in the above formula is that D is proportional to f. Thus, if we close the diaphragm by two stops, D doubles. The above formula, of course, does not take diffraction into account. In the real world, diffraction begins to visibly degrade the image at around f/11 to f/22. If you need the highest possible sharpness at the expense of depth of field, do not stop down more than f/8 to f/16 (you may need to experiment with your lens to find the smallest aperture that still gives the highest sharpness). On the other hand, if you need depth of field more than maximum sharpness on the plane of focus, feel free to stop down beyond this point. If you know that you will use a picture only at a low resolution on a web site, for instance, you do not need to be overly concerned about diffraction.

Hyperfocal distance

When avery low reproduction ratio is used, the subject is far from the camera, and the depth of field extends further to the back of the subject than it does in front of it. In this situation, it is useful to use the hyperfocal distance, which is computed as: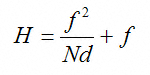

where H is the hyperfocal distance, f is the focal length, and N expresses the aperture as N = 2^i/2 , where i = 1, 2, 3,... for f/1.4, f/2, f/2.8, etc. From H one computes how much the depth of focus extends in front (D_n) and at the back (D_f) of the distance of focus of the lens (s):

The hyperfocal distance is particularly useful in landscape photography. In this type of photography, a high depth of focus, encompassing everything in sight, is usually desirable. You might be tempted to achieve this by focusing at infinity and closing the diaphragm as much as possible, in order to extend the depth of field to objects in the foreground. However, this is not a reasonable solution. In fact, by doing this you would be wasting more than half of the actual depth of field (i.e., the one located beyond infinity). In addition, you would be introducing diffraction as the diaphragm approaches its minimum diameter, which makes everything slightly fuzzy. You would also be forcing a high exposure time, which might spoil the picture if wind is blowing and leaves are moving. As an alternative, you might think of focusing in the foreground and stopping down. Also this is not optimal, because part of the depth of field (i.e., the one located in front of the foreground) is wasted. So, it appears that you must set the focus somewhere between the foreground and background in order to get both in focus. But where exactly should you focus, and what aperture should you use? As for the latter, you should use an aperture that is sufficiently, but not excessively small.

Using the hyperfocal distance eliminates the guesswork. You can build a table of hyperfocal values (obtained from the above formulas), using the focal length of your lens and a circle of confusion appropriate to your camera. For instance, assuming a focal length of 300 mm and a circle of confusion of 0.02 mm, we obtain the table (values are approximated for ease of presentation):

If we know, for instance, that we need a depth of focus ranging from 100 m (i.e., D_n) to infinity with our 300 mm lens, using the above table we set the focus at 200 m (i.e., H) as indicated on the focus scale of the lens, and the diaphragm at f/22 (or a higher value). With a 60 mm lens, the table becomes:

This table tells us, for instance, that with the diaphragm set at f/11 and the focus ring at 16 m, everything between 8 m and infinity will be in focus. Similarly, a 20 mm at f/4, focused at 5 m, will render everything sharp between  2.5 m and infinity, and a 10 mm at f/4, focused at 1.2 m, will render everything sharp between 60 cm and infinity.

You can use the same method to compute the depth of field of a lens focused at close-up or macro range. In this case, of course, the far distance is not infinity, and the goal is making the best use of the available depth of field. For instance, a 60 mm lens focused at30 cm will have a depth of field ranging between 29.1 and 30.5 cm at f/22, and between 29.4 and 30.2 cm at f/8.

If you know you are going to use hyperfocSal distances, youmay compute and print out tables of appropriate values for the lenses youown, and store them in your camera bag. Some photographers attach thesetables to the lens caps, to make sure that a table will stay with theappropriate lens. The external surface of the lens shade is another feasibleplace to attach a table. Through a web search you may also find sites thatoffer tables and graphs of hyperfocal values, sliding calculators that youcan cut out of cardboard, Excel spreadsheets and free software for thispurpose. Commercial tables and software are also available. You may evenpurchase a T-shirt with a hyperfocal table (printed upside-down, so you caneasily read it while wearing the T-shirt) on the Nikoniansweb site.

Depth of field is a special problem in close-up, macro photography and photomacrography.This topic is discussedin detail here.